Outline
Calculus
Sketches for calculus.
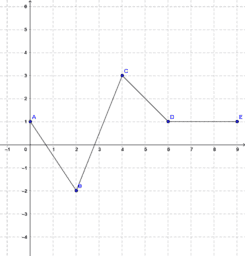
Table of Contents
Derivatives
- Derivative of a linear piecewise function
- Drawing Derivatives
- Tangent Zoom
- Slope and Tangent Lines
- Relating Tangent values to the derivative function
- Calculus of Trigonometry
- Function Composition
- Chain Rule
- Open Box
- Minimizing the total time spent on a hike
- Two Shapes from a Wire
- Leaving Fargo
- Taylor Approximation
- Baseball: Distance between two players
- Derivative Guess
- Derivative of Sine
- Integration by Substitution
- Related Rates (Rolling Carts Problem)
- Related Rates
- Finding Rate of Change at Points
- Off Road
- Cost of a Can
- USPS Package
- Filling Flasks
Integrals
Limits