Yazd
domes
Curvature and width of a dome varies with its height. You can’t place the same number of congruent stars at every height. For this an ingenious technique was established that could be applied as well inside as outside of domes.
- In the apex of the dome a star was placed, often12- or 16-pointed.
- From apex to base the dome was divided into sectors of curved triangles, as many as the number of points of the stars at the apex. These curves triangles were used as unit repeat for the dome.
- The curved triangles were filled in with stars, depending on the curvature of the dome. Doing so one could keep the segments on the stars constant and creating bigger and smaller stars.
- On each row figures were created to connect the stars. The aim was to use as little different figures as possible and to avoid angles that distort the harmony of the dome.
interiour dome of the Friday Mosque Yazd (Iran - 1375)
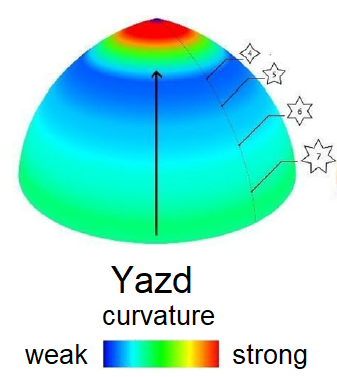
The interior dome of the Friday Mosque in Yazd show a solution with rows of stars. In the apex there’s a 16-pointed star. Towards the base there are rows of 16 stars, subsequently 4-, 5-, 6 and 7-pointed.
In the picture below you can see how the decrease of the curvature of the dome matches with less pointed stars.
The division of the dome into 16 sectors matches with a 16-pointed star in the apex of the dome.
