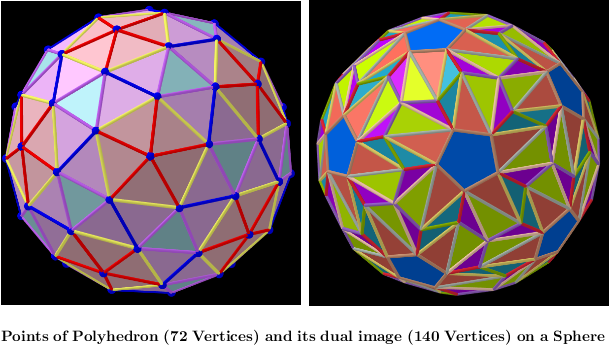
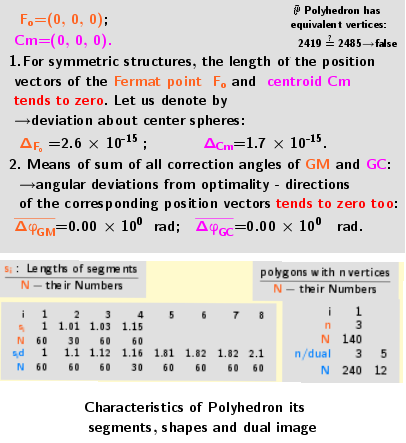
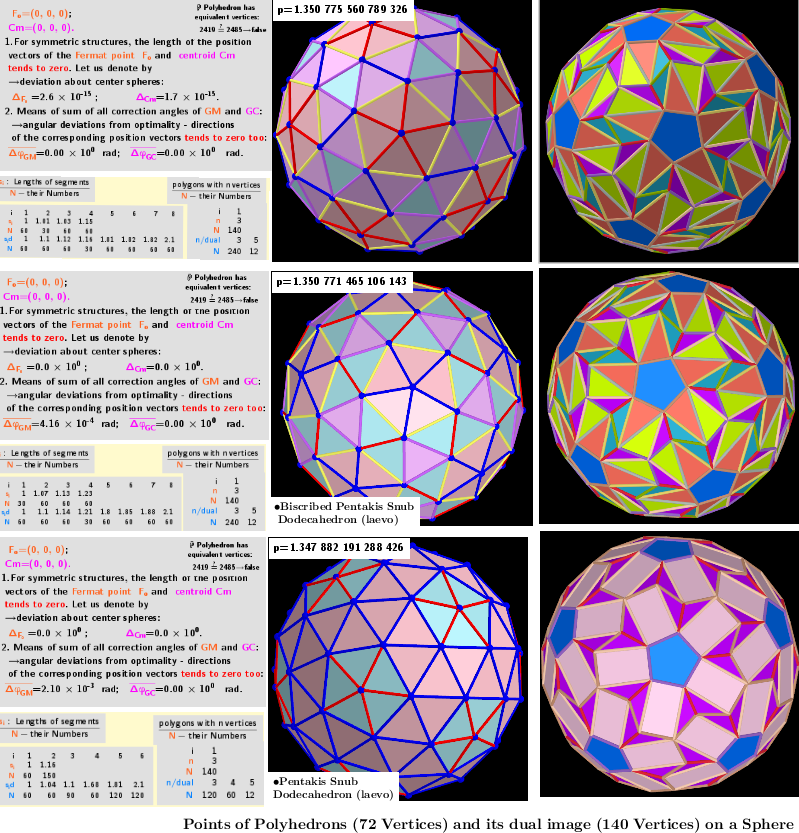
Coloring of edges and faces of a polyhedron(n=72) extreme distribution and its dual image.
Is considered as an example of the distribution of n=72 points on the surface of a sphere. In the applet, you can explore their extreme distribution. Two known distributions:
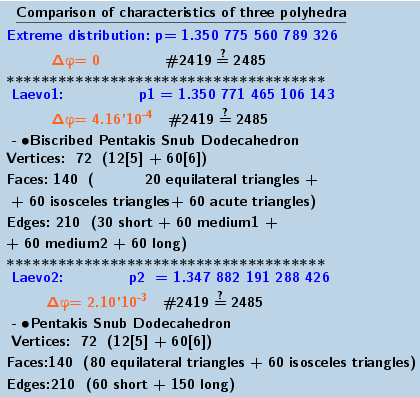
Biscribed Pentakis Snub Dodecahedron (laevo),
Pentakis Snub Dodecahedron (laevo).
-are not extreme(in terms of the extreme value of the Distance Sum - sum of their mutual distances).
Coloring of edges and faces of these polyhedra in applets:
Extreme distribution
Biscribed Pentakis Snub Dodecahedron (laevo)
Pentakis Snub Dodecahedron (laevo) .