Lab 1 Incenters, Circumcenters, and Centroids.
Enter your name:
Enter your partner's name:
Do Now:
1. Construct point P such that it is equidistant form the sides of ABC. Your construction must pass the "drag test." That is, if I click and drag any of the points, A, B, or C, your point must remain equidistant from the sides as the angle changes shape. You are not limited to compass and straightedge construction techniques (you may use any of the GeoGebra tools if you think they will make your work easier).
2. In the sketch above.
a. Construct a segment, PQ, the length of which represents the distance from point P to side BA.
b. Then construct segment PR, the length of which represents the distance from point P to side BC.
In constructions a and b, you may use the perpendicular tool: 
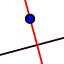the point of intersection tool 
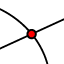, the segment tool 
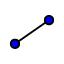, and the angle measure tool to show the relevant right angles 
.
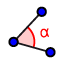c. Measure PR and PQ using the distance tool.
.
the point of intersection tool , the segment tool , and the angle measure tool to show the relevant right angles .
c. Measure PR and PQ using the distance tool..Group Work:
3. In the sketch below, construct a point that is equidistant from the sides of the triangle (you may use the tools listed above).
This point is called the Incenter of the triangle.
4. Construct the segments that could be measured to find the distance from the incenter to each side of the triangle.
5. Measure each segment using the distance tool.
6. In the sketch above, construct a circle centered at the incenter, and which has a radius equal to the length of the segments created in step 4.
7. The circle drawn in step 6 is said to be Inscribed in triangle ABC. How many times does your inscribed circle intersect each side of the triangle?
8. On the sketch below, construct a point which is equidistant from the vertices of triangle DEF. You can use any tool you wish. But, you will likely find the following tools helpful:
Midpoint Tool 
, Perpendicular Tool , point of intersection tool and the distance measurement tool .
Your constructed point must pass the "drag-test."
, Perpendicular Tool , point of intersection tool and the distance measurement tool .
Your constructed point must pass the "drag-test."9. State the theorem you used in your construction above and explained how it applied:
10. Construct a circle which intersects each vertex of triangle DEF above. This circle is said to be Circumscribed about triangle DEF. Alternately, triangle DEF is said to be inscribed in the circle.
11. Construct all three medians in triangle XYZ, below.
12. What do you observe about these medians?
13. Measure the medians, and each of the partitioned segments on the medians, using the distance tool. What do you notice about the measures? Is there a consistent mathematical relationship between any of them?
When three lines meat at one point, the lines are said to be concurrent. The point at which the lines meet is said to be their point of concurrency of the lines.
The three points of concurrency you discovered today are called the incenter, the circumcenter, and the centroid of a triangle.
15. How do you locate the incenter of a triangle and what are its properties?
16. How do you locate the circumcenter of a triangle and what are its properties?
17. How do you locate the centroid of a triangle and what are its properties?
18. Construct all three altitudes in triangle LMN, below.
19. The point of concurrency you discovered above is called the orthocenter of the triangle. On its own, it has no spectacular features. But, it is related to some of the other points of concurrency. On the triangle below:
a: construct the orthocenter, the centroid, and the circumcenter. Right click on each point, and rename it accordingly.
b: Hide the lines necessary for each construction.
c: Right-click one of the vertices of the triangle and animate the point. If this option is not available, simply drag the point around the screen and observe what happens to the points you created in part a.
From Handout 5_2 (homework). 1st problem.
Oliver broke the fundamental rule of geometry class, and constructed a circle without first marking its center (his construction is “pointless”, ha-ha. . . wait. There are a lot of problems with that joke. Okay, moving on). When he lifted his compass, he realized he had lost track of the precise location of the center. Using a compass and straightedge, help Oliver by locating the center (leave all arcs shown necessary for your construction).