Examples

Author:: P Porras

Example 1.

An 80-kg football player going north at 3 m/s tackled by a 100-kg player going east at 2 m/s. After the impact, which way are they going and how fast?

Solution 1.

The information about players enables us to draw a vector format of the situation:

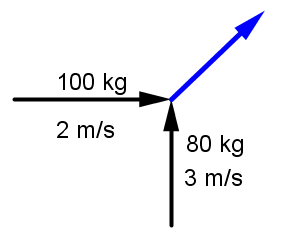
Eastward momentum is

northward momentum is

and the mass after the collision ("they continue together") on m_t = 180 kg. After the collision the momentum of the system stays constant. Thus, the speeds can be obtained the combined mass:

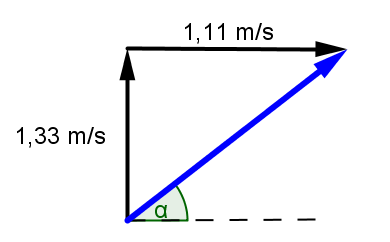
The final speed after the collision is obtained with Pythagoras:

The system continues at the angle of 50° from east to north -direction, as

Example 2.

There are three points A, B and C. Let us define

and

. There is a point P on the line segment AB so that it divides the line segment in a ratio 3:4. Define

with known

and

Solution 2.

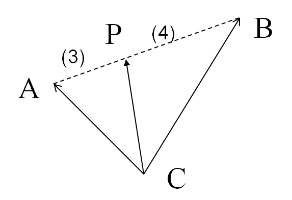
If ratio is thought as a distance, it could mean, for example, that the distance between A and P is 3 cm and between B and P 4 cm. Thus, the total distance between A and B is 7 cm and from A to P is only 3/7 of AB:

Example 3

Let

and

be known as vectors. There is a point on the extension of line segment AB so that

Define a vector

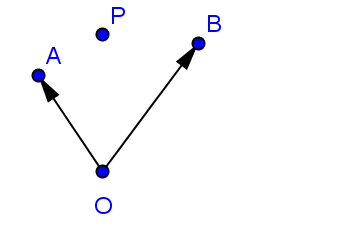
Solution 3.

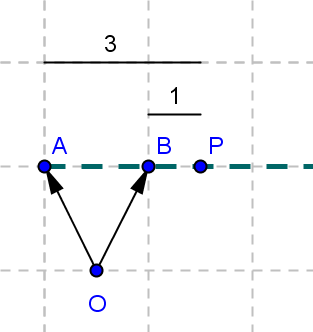
Because the point P is on the extension of the line segment AB, it cannot situate between the points. The ratio tells us that the point P is closer to the point B (in this case on the right side). Now, the line segment AB is of the line segment AP, so BP is half of the line segment AB.